HySafe Wiki | BRHS / Interaction Of Blast Wave With Structure

The effects from an explosion, which have an impact on structures, are pressure changes (blast wave) and air movement (“explosion wind”) as well as thermal radiation and flying missiles. Only a third of the chemical explosion energy is involved in the generation of the detonation blast wave; the other two thirds are released much slower during the subsequent mixing and burning of the detonation products with the air (FEMA426:2003). In general, structural responses are highly dynamic, highly inelastic, and highly interactive. The mechanical effect of a blast wave is determined by the overpressure and the duration of the positive phase.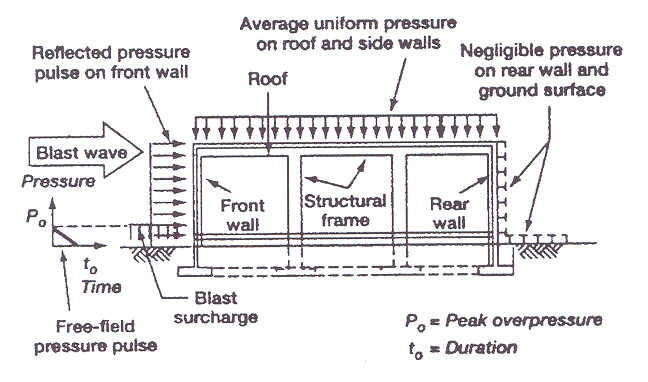

Fig. 1: Simplified blast loading diagram on a
rectangular building ([[http://hysafe.org/wiki/BRHS/Interaction?action=bibentry&bibfile=DB&bibref=BenteftifaCA:1995 | BenteftifaCA:1995)]]

The blast parameters are dependent on the distance between structure and blast center. At close distances, the target is exposed to a high-intensity pressure load over a localized region; at greater distances, the load is reduced, but covers a larger surface area. The diagram in Fig. 1 describes the pressure load on a rectangular structure.

The dynamic interaction of a blast wave with the structure depends on the pressure-time history, i.e., rise time and duration of positive phase and peak pressure or the impulse (which is the time integral of the pressure). Two phases are distinguished:

	1. The initial “diffraction loading”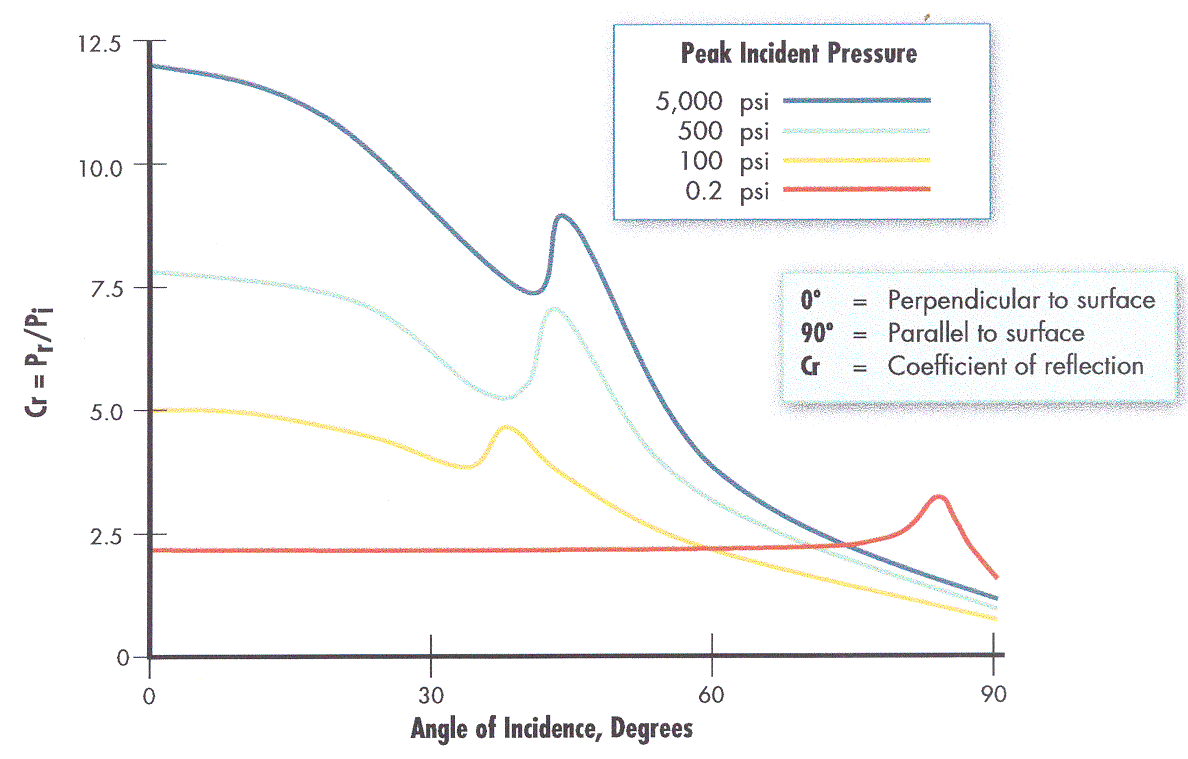

Fig. 2: Reflected pressure coefficient as a
function of the incidence angle (100 psi = 2.26 MPa)
([[http://hysafe.org/wiki/BRHS/Interaction?action=bibentry&bibfile=DB&bibref=FEMA426:2003 | FEMA426:2003) ]]

Diffraction loading is given by forces resulting from direct and reflected pressures during the initial phase. Reflection of the pressure wave at the front side amplifies the incident peak pressure with a normally reflected wave to represent an upper limit. Also density and temperature of the reflected wave are increased compared to the incident wave. The flow around the obstacle determines the further pressure development at the front and at the back side. The net horizontal loading is that on the front minus that on the back face. The reflection coefficient, i.e., the ratio between reflected and incident overpressure, is dependent on the blast wave type (pressure or shock wave), its intensity, and on the incident angle. For a pressure wave, this coefficient can have a value up to about 3 depending on the incident angle (the more usual case will have an oblique incidence). For a shock wave, it can be in the range of 2 to 8 and even higher for explosives (Fig. 2). Dynamic loads of fast transient pressures are imposed, if the combustion energy is inhomogeneously distributed, and are specific to the structure geometry.

  	2. The  “drag loading”

After the diffraction phase is completed, the structure is subjected to a “stagnation pressure”. The distance of the incident wave when interacting with the structure, causes major pressure differences developing from the edges of the structure. Resulting from this rarefaction wave, pressures decrease. During this so-called drag phase, strong transient winds (explosion wind) with flow velocities of several 100 m/s are effective. Drag forces will particularly have an impact on smaller structures such as pipe work. Load duration during vapor cloud explosions may be long enough to be comparable with the time required for the dynamic response time of the structure. In case of large structures, the rarefaction from the edges is insignificant.

Confined Areas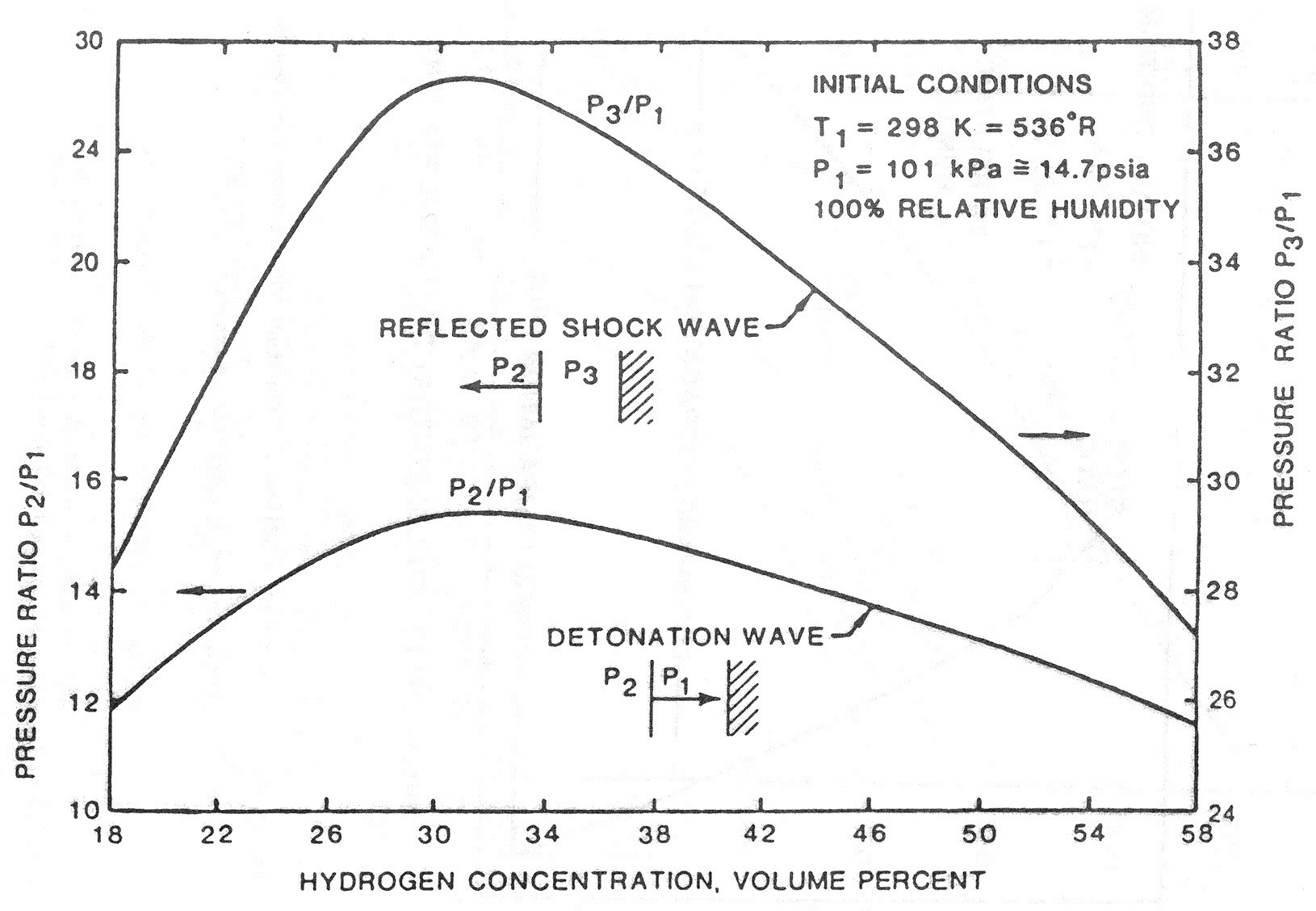

Fig. 3: Theoretical detonation pressure and
normally reflected detonation pressure
for hydrogen-air mixtures (IAEA 1990)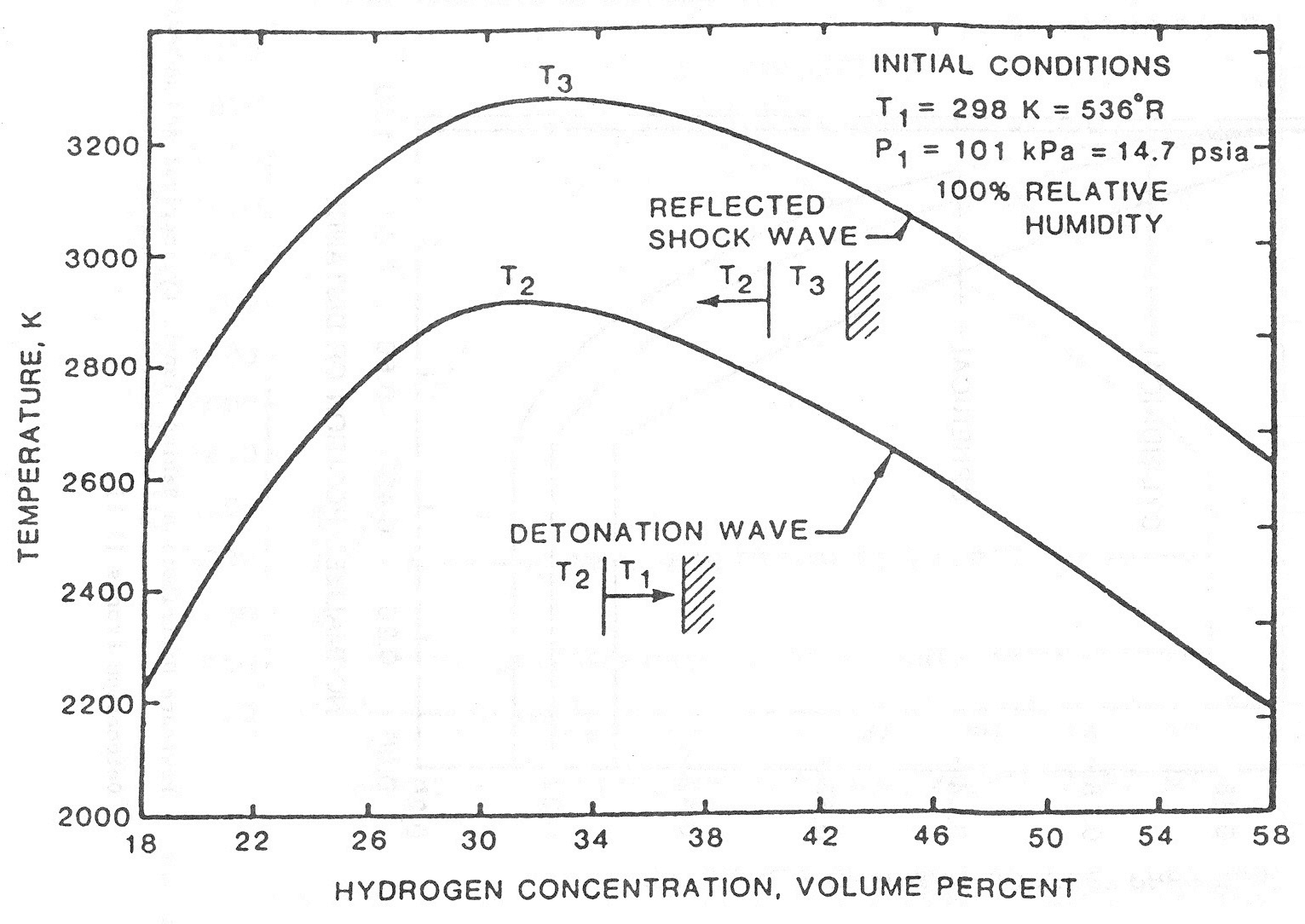

Fig. 4: Theoretical detonation temperature and
normally reflected detonation temperature
for hydrogen-air mixtures ([[http://hysafe.org/wiki/BRHS/Interaction?action=bibentry&bibfile=DB&bibref=IAEA:1990 | IAEA:1990)]]

In a confined or partially obstructed area, an explosion will create a structure loading, where two phases can be distinguished, the reflected blast loading followed by the gas loading phase. When the pressure wave hits the (rigid) wall, gases are brought to a rest and the wave is reflected. At normal incidence, the reflected shock wave further compresses the burnt gases increasing the pressure by about a factor of 2.3. The mixture of reflected pressure waves and deflected air flows are the result of reverberation of the initial high-pressure, short-duration reflected wave with the amplitude decaying with each reflection until eventually pressure levels out at gas pressure loading. The latter phase lasts longer, the less venting is available. The more complex the structure, the more difficult is the prediction of the critical conditions for mechanical failure for a given load history.

For a vented volume or a volume with weak surfaces, venting formula (NFPA68:2002), (prEN14994:2004) may be a tool in order to predict the pressure applied. In case of a detonation, the loading may be divided in two parts (USACE:1990), a shock pressure and a constant load.

Invalid BibTex Entry!

<< Throw of Debris and Missiles | Content | Methods to Determine Structural Responses >>