
|
BRHS /
Dependence of laminar burning velocity on mixture composition, pressure and temperature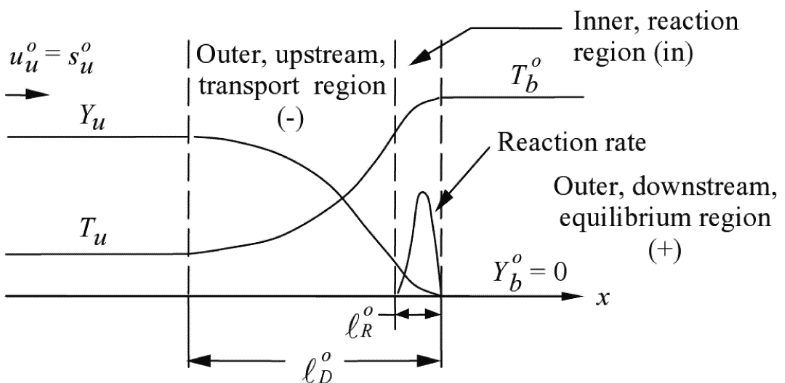 Figure 1. Temperature-, species- and reaction rate profile across the premixed flame structure. From Law (2006) (LawCK:2006a). The laminar burning velocity is known to depend on mixture composition, pressure and temperature. Many correlations have been proposed to describe this dependence. One way of deriving correlations for the effect of mixture composition, pressure and temperature rests on representing the kinetics mechanism by a single overall Arrhenius reaction where \nu_{\scriptsize \mathrm{F}} fuel molecules react with \nu_{\scriptsize \mathrm{Ox}} oxidiser molecules into \nu_{\scriptsize \mathrm{Pr}} product molecules: \nu_{\scriptstyle \mathrm{F}}\mathrm{F} + \nu_{\scriptstyle \mathrm{Ox}}\mathrm{Ox} \mathop{\rightarrow}_{\,}^{k} \nu_{\scriptstyle \mathrm{Pr}}\mathrm{Pr} \qquad \mathrm(1a)
with k denoting an overall reaction rate of order n = \nu_{\scriptstyle \mathrm{F}} + \nu_{\scriptstyle \mathrm{Ox}} defined as: k=A T^{m} \mathrm{exp}\left(-\frac{\mathrm{E_a}}{\mathrm{R}T}\right) \qquad \mathrm(1b)
so that \dot{\omega}_{\scriptstyle \mathrm{F}}=\frac{d\left[\mathrm{F}\right]}{dt}=-A T^{m} \mathrm{exp}\left(-\frac{\mathrm{E_a}}{\mathrm{R}T}\right)\left[\mathrm{F}\right]^{\scriptstyle \nu_{\scriptscriptstyle \mathrm{F}}}\left[\mathrm{Ox}\right]^{\scriptstyle \nu_{\scriptscriptstyle \mathrm{Ox}}} \qquad \mathrm(2a)
\dot{\omega}_{\scriptstyle \mathrm{Ox}}=\frac{d\left[\mathrm{Ox}\right]}{dt}=\frac{\nu_{\scriptstyle \mathrm{Ox}}}{\nu_{\scriptstyle \mathrm{F}}}\dot{\omega}_{\scriptstyle \mathrm{F}}= - \frac{\nu_{\scriptstyle \mathrm{Ox}}}{\nu_{\scriptstyle \mathrm{F}}} A T^{m} \mathrm{exp}\left(-\frac{\mathrm{E_a}}{\mathrm{R}T}\right)\left[\mathrm{F}\right]^{\scriptstyle \nu_{\scriptscriptstyle \mathrm{F}}}\left[\mathrm{Ox}\right]^{\scriptstyle \nu_{\scriptscriptstyle \mathrm{Ox}}} \qquad \mathrm(2b)
\dot{\omega}_{\scriptstyle \mathrm{Pr}}=\frac{d\left[\mathrm{Pr}\right]}{dt}=-\frac{\nu_{\scriptstyle \mathrm{Pr}}}{\nu_{\scriptstyle \mathrm{F}}}\dot{\omega}_{\scriptstyle \mathrm{F}}= + \frac{\nu_{\scriptstyle \mathrm{Pr}}}{\nu_{\scriptstyle \mathrm{F}}} A T^{m} \mathrm{exp}\left(-\frac{\mathrm{E_a}}{\mathrm{R}T}\right)\left[\mathrm{F}\right]^{\scriptstyle \nu_{\scriptscriptstyle \mathrm{F}}}\left[\mathrm{Ox}\right]^{\scriptstyle \nu_{\scriptscriptstyle \mathrm{Ox}}} \qquad \mathrm(2c)
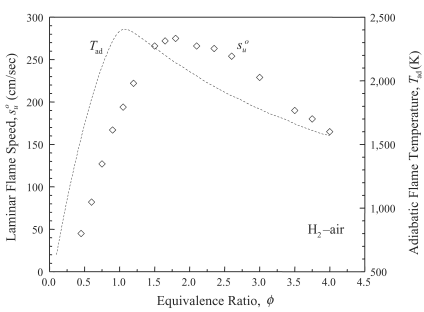 Figure 2. Calculated adiabatic flame temperatures and measured laminar flame speeds of atmospheric hydrogen–air mixtures. From Law (2006) (LawCK:2006a). The overall reaction is then used to solve the following mass and heat conservation equations for the situation depicted by Figure 1 (p247 of Law (2006) (LawCK:2006a)): \rho_u {S_u^{\circ}}_{L}\frac{dY_{\scriptstyle \mathrm{F}}}{dx} - \rho \mathcal{D}\frac{d^2Y_{\scriptstyle \mathrm{F}}}{dx^2} =-\dot{\omega}_{\scriptstyle \mathrm{F}} \qquad \mathrm(3a)
\rho_u {S_u^{\circ}}_{L}\hat{C}_{\scriptstyle P}\frac{dT}{dx} - \lambda\frac{d^2T}{dx^2} =\Delta_{\scriptstyle \mathrm{R}}\hat{H}\dot{\omega}_{\scriptstyle \mathrm{F}} \qquad \mathrm(3b)
The influence of mixture composition on the laminar burning velocity is mainly via the effect it has on the adiabatic flame temperature T_f^{\scriptsize \text{ad}} (page 275-276 of Law (2006) (LawCK:2006a)). Figure 2 shows that the laminar burning velocity and T_f^{\scriptsize \text{ad}} show similar responses as a function of the equivalence ratio (although the peak of the former is offset to \phi=1.75). A correlation derscribing the dependence of the laminar burning velocity on T_f, and hence on \phi is given by Law (2006) (LawCK:2006a)): \frac{\left(\rho{S_u}_{L}\right)}{\left(\rho{S_u}_{L}\right)^{\circ}} = \left(\frac{T_f}{T_f^{\circ}}\right)^2 \mathrm{exp}\left[-\frac{\mathrm{Ea}}{2\mathrm{R}}\left(\frac{1}{T_f}-\frac{1}{T_f^{\circ}}\right)\right] \qquad (4)
where the suffix ^{\circ} denotes reference conditions of pressure, temperature and composition. An extensive comparison of laminar burning velocities of hydrogen mixtures as a function of equivalence ratio is given in Figure 3. The data in this figure are from Takahashi, Mizomoto & Ikai (1983) (TakahashiF:1983); Dowdy, Smith, Taylor & Williams (1990) (DowdyDR:1990); Koroll, Kumar & Bowles (1993) (KorollGW:1993); Vagelopoulos & Egolfopoulos (1995); (VagelopoulosCM:1995); Iijima & Takeno (1986) (IijimaT:1986); Wu & Law (1984) (WuCK:1984); Egolfopoulos & Law (1990) (EgolfopoulosFN:1990); Lamoureux, Djebaili-Chaumeix & Paillard (LamoureuxN:2002); Lamoureux, Djebaili-Chaumeix & Paillard (LamoureuxN:2002); Kwon & Faeth (2001) (KwonOC:2001); Law C.K. (1993) (LawCK:1993); Aung, Hassan & Faeth (1997) (AungKT:1997); Tse, Zhu & Law (2000) (TseSD:2000), and, Marinov, Westbrook & Pitz (1996) (MarinovNM:1996). 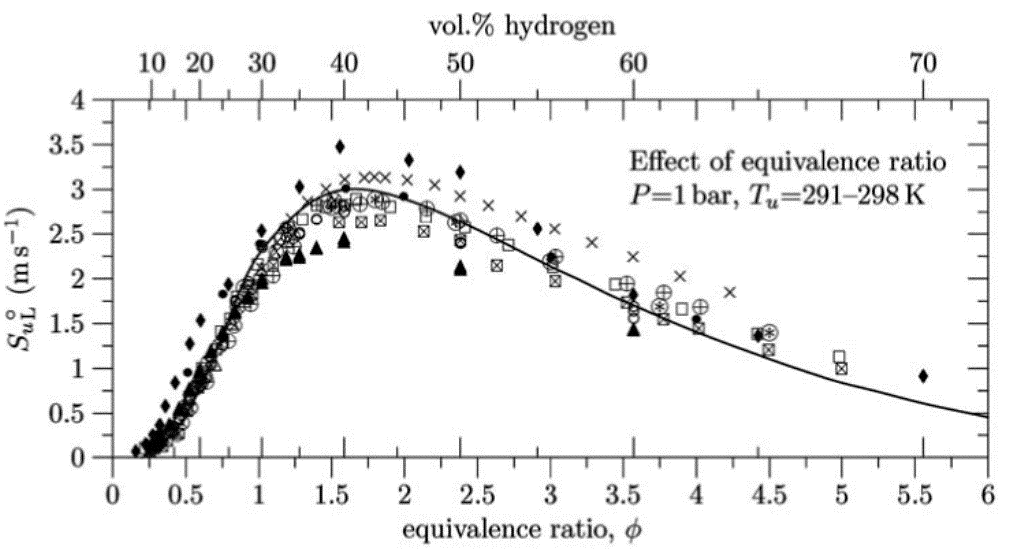 Figure 3. Dependence of the laminar burning velocity on equivalence ratio at normal pressure and temperatures ranging between 293 and 298 K. From Dahoe (2005) (DahoeAE:2005). Figures 2 and 3 indicate that the safety critical combustion properties of hydrogen-air mixtures at atmospheric pressure and room temperature are as follows:
Liu & MacFarlane (1983) (LiuDDS:1983) studied the burning velocity of hydrogen-air mixtures diluted by steam. The steam concentration was varied from 0 vol.% to 15 vol.%. An empirical correlation was developed to describe the \mathrm{H_2}-air-steam burning velocity as a function of the hydrogen concentration, steam concentration and temperature: {S_u}_{L} = B T_u^C \mathrm{exp}\left({D\,x_{\scriptstyle\mathrm{H_{\scriptscriptstyle 2}O}} }\right) \qquad \mathrm(5)
 Figure 4. Laminar burning velocity of hydrogen air mixtures at room temperature and atmoshpheric measured by various methods. Solid line: computed values for spherical flame propagation. Dotted line: correlation equation of Liu & MacFarlane (1983) (LiuDDS:1983). where {S_u}_{L} denotes laminar burning velocity, B, C, D are coefficients, T_u the temperature of of the unburned gas, x the volumetric fraction of the \mathrm{H_{2}O} . The coefficients for this correlation are given in Table 1. The reported root mean square deviation of the correlation is 0.189 m/s. These authors suggested that the correlation may be applied in the whole range of flammable hydrogen concentrations (5-75 vol.%) volume and the steam concentration range (0-25 vol.%) in the experimental temperature range from 293-523K. The correspondence between this correlation and experimental data is illustrated in Figure 4. Table 1. Coefficients in the burning velocity correlation (5).
The combustion of \mathrm{H_2}-air-steam mixtures was also studied by Koroll, Kumar & Bowles (KorollGW:1993) in the steam concentration range from 12 vol.% to 42 vol.% These authors developed a correlation for the burning velocity of \mathrm{H_2}-air-diluent mixtures and \mathrm{H_2}-air-steam/diluent mixtures, which is applicable to the \mathrm{H_2} concentration range from 9 vol.% to 70 vol.%. Studies of the laminar burning velocity of \mathrm{H_2}-air-steam/diluent mixtures were also undertaken by Lamoureux, Djebaili-Chaumeix & Paillard (LamoureuxN:2002), Tse, Zhu & Law (2000) (TseSD:2000), and Kwon & Faeth (2001) (KwonOC:2001). 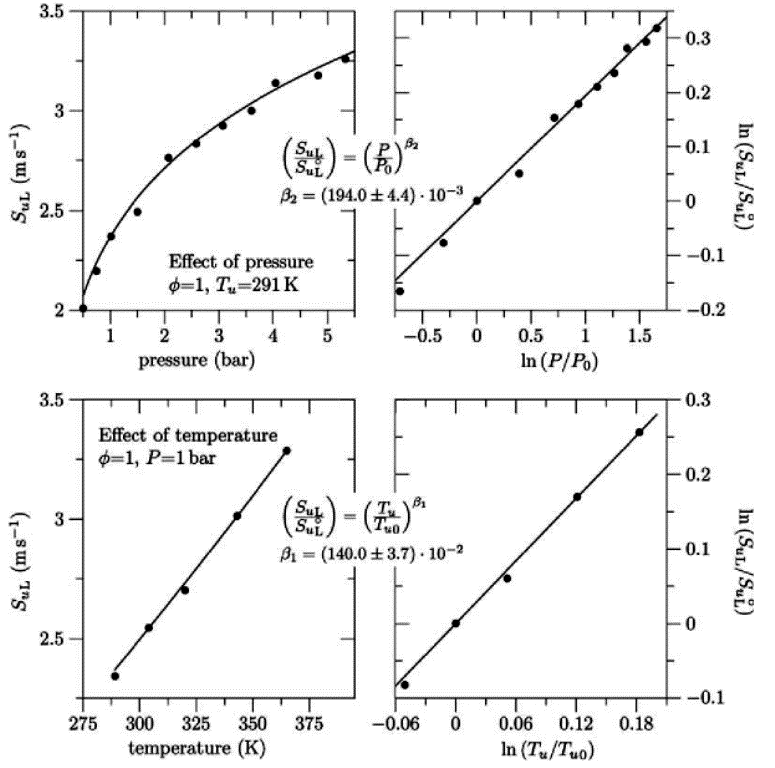 Figure 5. Effect of pressure (upper part) and temperature (lower-part) on the laminar burning velocity of stoichiometric hydrogen–air mixtures From Dahoe (2005) (DahoeAE:2005). The data points in this figure are from Iijima & Takeno (1986) (IijimaT:1986). The same approach based on an assumed overall reaction which led to equation (4) is also used to obtain expressions for the effect of temperature and pressure on the laminar burning velocity (see Glassman (1996) (GlassmanI:1996), Law (2006) (LawCK:2006a), and, Kuo (2005) (KuoKK:2005)). Correlations for the effect of initial temperature and pressure obtained in this manner take the form (see Figure 5) \frac{{S_u}_{L}}{{S_u}_{L}^{\circ}} \propto \left(\frac{{T_u}}{{T_u}_0}\right)^{\scriptstyle \beta_1} \quad \mathrm(6a)
\frac{{S_u}_{L}}{{S_u}_{L}^{\circ}} \propto \left(\frac{{P}}{P_0}\right)^{\scriptstyle\frac{\scriptstyle n-2}{\scriptstyle 2}} \propto \left(\frac{{P}}{P_0}\right)^{\scriptstyle \beta_2} \quad \mathrm(6b)
where n denotes the overall reaction order of equation (1a), and, \beta_1 and \beta_2 respectively denote the temperature and pressure exponent. For modeling purposes involving simultaneous changes in temperature and pressure the above equations are combined as follows: \frac{{S_u}_{L}}{{S_u}_{L}^{\circ}} \propto \left(\frac{{T_u}}{{T_u}_0}\right)^{\scriptstyle \beta_1} \left(\frac{{P}}{P_0}\right)^{\scriptstyle \beta_2} \quad \mathrm(6c)
The value of \beta_1 and \beta_2 depends on the mixture composition (see Table 2). This implies that the overall reaction order n of equation (1a) also depends on the mixture composition. Table 2. Temperature and pressure exponents, \beta_1 and \beta_2, for \mathrm{H_2}-air laminar burning velocity (P_0 = 1 atm, {T_u}_0 = 298K). After Babkin (2003) (BabkinVS:2003).
* - values in brackets are interpolated or extrapolated, ** - values determined in the range p=0.25-1.0 atm. In the range of \mathrm{H_2} concentrations of 60-70 vol.% \beta_2 = (-0.4) to (-0.1) may be expected, (GrumerJ:1959). A particular issue with equation (6c) is that, when the reactant mixture composition remains the same, it does not take the effect of changes in the flame temperature due to variations in the initial temperature and pressure into account. While the effect of initial temperature on the flame temperature is small (see page 501 of Kuo (2005) (KuoKK:2005)), changes in the latter caused by variations in the pressure must be taken into account. For this reason there have also been attempts to derive more comprhensive expressions, such that these dependencies are expressed in terms of the pre-exponential temperature exponent m, the overall reaction order n, and the activation energy \mathrm{Ea} of equation (1c). Such attempts (e.g. Dahoe & de Goey (2003) (DahoeAE:2003)) have resulted in expressions for the laminar burning velocity and laminar flame thickness: \frac{{S_u}_{L}}{{S_u}_{L}^{\circ}} = \sqrt{\frac{\lambda(T_u)}{\lambda({T_u}_0)}}\frac{T_u}{{T_u}_0}\left(\frac{{P}}{P_0}\right)^{\scriptstyle (n-2)/2}\left(\frac{{T_f}}{T_f^{\circ}}\right)^{\scriptstyle -(n/2)}\left(\frac{{T_f}}{T_f^{\circ}}\right)^{\scriptstyle m/2}\mathrm{exp}\left[-\frac{\mathrm{Ea}}{2\mathrm{R}}\left(\frac{1}{T_f}-\frac{1}{T_f^{\circ}}\right)\right] \quad \mathrm(7a)
\frac{\delta_{L}}{\delta_{L}^{\circ}} = \sqrt{\frac{\lambda(T_u)}{\lambda({T_u}_0)}}\left(\frac{{P}}{P_0}\right)^{\scriptstyle -(n/2)}\left(\frac{{T_f}}{T_f^{\circ}}\right)^{\scriptstyle n/2}\left(\frac{{T_f}}{T_f^{\circ}}\right)^{\scriptstyle -(m/2)}\mathrm{exp}\left[+\frac{\mathrm{Ea}}{2\mathrm{R}}\left(\frac{1}{T_f}-\frac{1}{T_f^{\circ}}\right)\right] \quad \mathrm(7b)
where \lambda denotes the thermal conductivity. Aung K.T., Hassan M.I. and Faeth G.M. (1997) Flame stretch interactions of laminar premixed hydrogen/air flames at normal temperature and pressure. Combustion and Flame, 109:1-24.(BibTeX) << Laminar burning velocity and laminar flame thickness | Content | Flame stretch and effect of flame curvature, Markstein lengths >> |